Next: Derivation of the
Key equations and physical effects governing plasma behavior
- Maxwell's equations for the microscopic electric (E) and
magnetic (B) fields
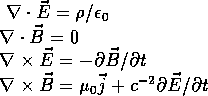
- Electrostatic potential definition and Poisson's equation
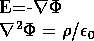
- Lorentz force on a particle of charge q
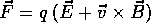
- Plasmas Will Try to Reach Thermodynamic Equilibrium
Neglecting boundary effects, equilibrium is represented by the Maxwell--Boltzmann or Maxwellian distribution of particles in energy,
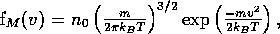
where
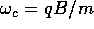is the average charge density and
. The latter value is given because it is often convenient to measure plasma energies and temperatures in eV rather than K (1 eV=11,604 K).
- Plasmas are Dynamic Entities
Electrons are particulary mobile. For example, the typical velocities for ions and electrons in a hydrogen plasma are
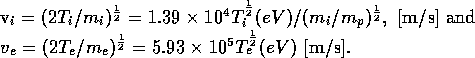
- Debye Shielding
An important consequence of the high plasma mobility is Debye shielding, in which electrons tend to cluster around negative density fluctuations and to avoid positive density fluctuations. The Debye length, or Debye screening distance, gives an estimate of the extent of the influence of a charge fluctuation. It plays an extremely important role in many problems. The Debye length is given by
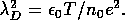
Click here for a derivation of the Debye length.
- Plasma Parameter
The number of particles, N, in a Debye sphere (sphere with radius equal to the Debye length) must satisfy N>>1 in order for there to be statistical significance to the Debye shielding mechanism:
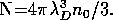
In general, the condition N>>1 is necessary for collective effects to be important.